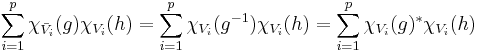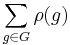Representation theory of finite groups
In mathematics, representation theory is a technique for analyzing abstract groups in terms of groups of linear transformations. See the article on group representations for an introduction. This article discusses the representation theory of groups that have a finite number of elements.
Basic definitions
All the linear representations in this article are finite dimensional and assumed to be complex unless otherwise stated. A representation of G is a group homomorphism ρ:G → GL(n,C) from G to the general linear group GL(n,C). Thus to specify a representation, we just assign a square matrix to each element of the group, in such a way that the matrices behave in the same way as the group elements when multiplied together.
We say that ρ is a real representation of G if the matrices are real. In other words if ρ(G) ⊂ GL(n,R).
Other formulations
A representation ρ: G → GL(n,C) defines a group action of G on the vector space Cn. Moreover this action completely determines ρ. Hence to specify a representation it is enough to specify how it acts on its representing vector space.
Alternatively, the action of a group G on a complex vector space V induces a left action of group algebra C[G] on the vector space V, and vice-versa. Hence representations are equivalent to left C[G]-modules.
The group algebra C[G] is a |G|-dimensional algebra over the complex numbers, on which G acts. (See Peter–Weyl for the case of compact groups.) In fact C[G] is a representation for G×G. More specifically, if g1 and g2 are elements of G and h is an element of C[G] corresponding to the element h of G,
- (g1,g2)[h]=g1 h g2-1.
C[G] can also be considered as a representation of G in three different ways:
- Conjugation: g[h] = g h g−1
- As a left action: g[h] = g h (a regular representation)
- As a right action: g[h] = h g−1 (also);
these are all to be 'found' inside the G×G action.
Example
For many groups it is entirely natural to represent the group through matrices. Consider for example the dihedral group D4 of symmetries of a square. This is generated by the two reflection matrices
Here m is a reflection that maps (x,y) to (− x,y), while n maps (x,y) to (y,x). Multiplying these matrices together creates a set of 8 matrices that form the group. As discussed above, we can either think of the representation in terms of the matrices, or in terms of the action on the two-dimensional vector space (x,y).
This representation is faithful - that is, there is a one-to-one correspondence between the matrices and the elements of the group. It is also irreducible, because there is no subspace of (x,y) that is invariant under the action of the group.
Discrete Fourier transform
If G is a finite cyclic group, then its representation theory is called the discrete Fourier transform; this example is central to digital signal processing.
All irreducible representations are 1-dimensional (characters), and correspond to sending a generator of G to a root of unity, not necessarily primitive (the trivial representation sends a generator to 1, for instance).
A function on G is called the time domain representation of the function, while the corresponding expression in terms of characters is called the frequency domain representation of the function: changing from the time domain description to the frequency domain description is called the discrete Fourier transform, and the opposite direction is called the inverse discrete Fourier transform.
The character table, which in this case is the matrix of the transform, is the DFT matrix, which is, up to normalization factor, the Vandermonde matrix for the nth roots of unity; the order of rows and columns depends on a choice of generator and primitive root of unity.
The group of characters is isomorphic to G itself, but not naturally so, and is known as the dual group, 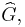 in the language of Pontryagin duality, and the original group G can be recovered as the double dual.
in the language of Pontryagin duality, and the original group G can be recovered as the double dual.
Abelian groups
More generally, any finite abelian group is a direct sum of finite cyclic groups (by the fundamental theorem of finitely generated abelian groups, though the decomposition is not unique in general), and thus the representation theory of finite abelian groups is completely described by that of finite cyclic groups, that is, by the discrete Fourier transform.
If an abelian group is expressed as a direct product, and the dual group likewise decomposed, and the elements of each sorted in lexicographic order, then the character table of the product group is the Kronecker product (tensor product) of the character tables for the two component groups, which is just a statement that the value of a product homomorphism on a product group is the product of the values: 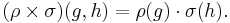
Morphisms between representations
Given two representations ρ: G → GL(n,C) and τ: G → GL(m,C) a morphism between ρ and τ is a linear map T : Cn → Cm so that for all g in G we have the following commuting relation: T ° ρ(g) = τ(g) ° T.
According to Schur's lemma, a non-zero morphism between two irreducible complex representations is invertible, and moreover, is given in matrix form as a scalar multiple of the identity matrix.
This result holds as the complex numbers are algebraically closed. For a counterexample over the real numbers, consider the two dimensional irreducible real representation of the cyclic group C4 = 〈x〉 given by:
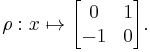
Then the matrix ![\left [ \begin{smallmatrix} 0 & 1 \\ -1 & 0 \end{smallmatrix} \right ]](/2012-wikipedia_en_all_nopic_01_2012/I/ad5a35cf765db7ca3a6cb389dfd8b8dc.png) defines an automorphism of ρ, which is clearly not a scalar multiple of the identity matrix.
defines an automorphism of ρ, which is clearly not a scalar multiple of the identity matrix.
Subrepresentations and irreducible representations
As noted earlier, a representation ρ defines an action on a vector space Cn. It may turn out that Cn has an invariant subspace V ⊂ Cn. The action of G is given by complex matrices and this in turn defines a new representation σ : G → GL(V). We call σ a subrepresentation of ρ. A representation without subrepresentations is called irreducible.
Constructing new representations from old
There are number of ways to combine representations to obtain new representations. Each of these methods involves the application of a construction from linear algebra to representation theory.
- Given two representations ρ1, ρ2 we may construct their direct sum ρ1 ⊕ ρ2 by (ρ1 ⊕ ρ2) (g)(v,w) = (ρ1(g)v, ρ2(g)w).
- The tensor representation of ρ1, ρ2 is defined by (ρ1 ⊗ ρ2) (v ⊗ w) = ρ1(v) ⊗ ρ2(w).
- Let ρ : G → GL(n,C) be a representation. Then ρ induces a representation ρ* on the dual of the vector space Hom(Cn,C). Let f : Cn → C be a linear functional. The representation ρ* is then defined by the rule ρ* (g) (f) = f(ρ(g)−1). The representation ρ* is called either the dual representation or the contragredient representation of ρ.
- Furthermore, if a representation ρ has a subrepresentation σ then the quotient of the representing vector spaces for ρ and σ has a well defined action of G on it. We call the resulting representation the quotient representation of ρ by σ.
Young tableau
For the symmetric groups, a graphical method exists to determine their finite representations that associates with each representation a Young tableau (also known as a Young diagram). The direct product of two representations may easily be decomposed into a direct sum of irreducible representation by a set of rules for the "direct product" of two Young diagrams. Each diagram also contains information about the dimension of the representation to which it corresponds. Young tableaux provide a far cleaner way of working with representations than the algebraic methods that underlie their use.
Applying Schur's lemma
Lemma. If f: A ⊗ B → C is a morphism of representations, then the corresponding linear transformation obtained by dualizing B is: f′: A → C ⊗ B* is also a morphism of representations. Similarly, if g: A → B ⊗C is a morphism of representations, dualizing it will give another morphism of representations g′: A ⊗ C* → B.
If ρ is an n-dimensional irreducible representation of G with the underlying vector space V, then we can define a G×G morphism of representations, for all g in G and x in V
- f: C[G] ⊗ (1G ⊗ V) → (V ⊗ 1G)
- f:(g ⊗ x) = ρ(g)[x]
where 1G is the trivial representation of G. This defines a G×G morphism of representations.
Now we use the above lemma and obtain the G×G morphism of representations
![f':\bar{V}\otimes V\rightarrow \overline{\mathbb{C}[G]}](/2012-wikipedia_en_all_nopic_01_2012/I/c04ef143b7d5a9397b08a323beb260d2.png) .
.
The dual representation of C[G] as a G×G-representation is equivalent to C[G]. An isomorphism is given if we define the contraction 〈g,h〉 = δgh. So, we end up with a G×G-morphism of representations
![f'':\bar{V}\otimes V\rightarrow \mathbb{C}[G]](/2012-wikipedia_en_all_nopic_01_2012/I/0810af8bdc9f78efbf56dc1c8a89f7cc.png) .
.
Then
for all x in  and y in V.
and y in V.
By Schur's lemma, the image of f″ is a G×G irreducible representation, which is therefore n×n dimensional, which also happens to be a subrepresentation of C[G] (f″ is nonzero).
This is n direct sum equivalent copies V. Note that if ρ1 and ρ2 are equivalent G-irreducible representations, the respective images of the intertwining matrices would give rise to the same G×G-irreducible representation of C[G].
Here, we use the fact that if f is a function over G, then
We convert C[G] into a Hilbert space by introducing the norm where 〈g,h〉 is 1 if g is h and zero otherwise. This is different from the 'contraction' given a couple of paragraphs back, in that this form is sesquilinear. This makes C[G] a unitary representation of G×G. In particular, we now have the concepts of orthogonal complement and orthogonality of subrepresentations.
In particular, if C[G] contains two inequivalent irreducible G×G subrepresentations, then both subrepresentations are orthogonal to each other. To see this, note that for every subspace of a Hilbert space, there exists a unique linear transformation from the Hilbert space to itself which maps points on the subspace to itself while mapping points on its orthogonal complement to zero. This is called the projection map. The projection map associated with the first irreducible representation is an intertwiner. Restricted to the second irreducible representation, it gives an intertwiner from the second irreducible representation to the first. Using Schur's lemma, this must be zero.
Now suppose A ⊗ B is a G×G-irreducible representation of C[G].
Note. The complex irreducible representations of G×H are always a direct product of a complex irreducible representation of G and a complex irreducible representation of H. This is not the case for real irreducible representations. As an example, there is a 2 dimensional real irreducible representation of the group C3 × C3 which transforms nontrivially under both copies of C3 but cannot be expressed as the direct product of two irreducible representations of C3.
This representation is also a G-representation (nA direct sum copies of B where nA is the dimension of A). If Y is an element of this representation (and hence also of C[G]) and X an element of its dual representation (which is a subrepresentation of the dual representation of C[G]), then
![=\sum_{g\in G}\langle X,g^{-1}\rangle gY=\sum_{g\in G}\langle X,g^{-1}\rangle (g,e)[Y]](/2012-wikipedia_en_all_nopic_01_2012/I/28785b066cb679c2aaa35d94dd1b4826.png)
where e is the identity of G. Though the f″ defined a couple of paragraphs back is only defined for G-irreducible representations, and though A ⊗ B is not a G-irreducible representation in general, we claim this argument could be made correct since A ⊗ B is simply the direct sum of copies of Bs, and we have shown that each copy all maps to the same G×G-irreducible subrepresentation of C[G], we have just showed that  as an irreducible G×G-subrepresentation of C[G] is contained in A ⊗ B as another irreducible G×G-subrepresentation of C[G]. Using Schur's lemma again, this means both irreducible representations are the same.
as an irreducible G×G-subrepresentation of C[G] is contained in A ⊗ B as another irreducible G×G-subrepresentation of C[G]. Using Schur's lemma again, this means both irreducible representations are the same.
Putting all of this together,
Theorem. C[G] ≅
where the sum is taken over the inequivalent G-irreducible representations V.
Corollary. If there are p inequivalent G-irreducible representations, Vi, each of dimension ni, then |G| = n12 + ... + np2.
Character theory
- Main article: Character theory
There is a mapping from G to the complex numbers for each representation called the character given by the trace of the linear transformation upon the representation generated by the element of G in question
- χρ(g)=Tr[ρ(g)].
All elements of G belonging to the same conjugacy class have the same character: in other words χρ is a class function on G. This follows from
- Tr[ρ(ghg-1)]=Tr[ρ(g)ρ(h)ρ(g)-1]=Tr[ρ(h)]
by the cyclic property of the trace of a matrix.
What are the characters of C[G]? Using the property that gh−1 is only the same as g if h = e, χC[G](g) is |G| if g=e and 0 otherwise.
The character of a direct sum of representations is simply the sum of their individual characters.
Putting all of this together,
with the Kronecker delta on the right hand side.
Repeat this, working with characters of G×G instead of characters, of G which I'll call Δ. Then, ΔC[G](g,h) is the number of elements k in G satisfying g k h−1 = k. This is equal to
where * denotes complex conjugation. After all, C[G] is a unitary representation and any subrepresentation of a finite unitary representation is another unitary representation; and all irreducible representations are (equivalent to) a subrepresentation of C[G].
Consider
![\sum_{h \in G}\Delta_{C[G]}((g,hkh^{-1}))](/2012-wikipedia_en_all_nopic_01_2012/I/d6e299e9e777d5dea27df119b2f9a823.png) .
.
This is |G| times the number of elements which commute with g; which is |G|2 divided by the size of the conjugacy class of g, if g and k belong to the same conjugacy class, but zero otherwise. Therefore, for each conjugacy class Ci of size mi, the characters are the same for each element of the conjugacy class and so we can just call χρ(Ci) by an abuse of notation). Then,
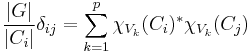 .
.
Note that
is a self-intertwiner (or invariant). This linear transformation, when applied to C[G] (as a representation of the second copy of G×G), would give as its image the 1-dimensional subrepresentation generated by
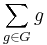 ;
;
which is obviously the trivial representation.
Since we know C[G] contains all irreducible representations up to equivalence and using Schur's lemma, we conclude that
for irreducible representations is zero if it's not the trivial irreducible representation; and it's of course |G|1 if the irreducible representation is trivial.
Given two irreducible representations Vi and Vj, we can construct a G-representation
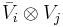 ,
,
this time not as a G×G representation but an ordinary G-representation. See direct product of representations. Then,
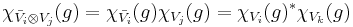 .
.
It can be shown that any irreducible representation can be turned into a unitary irreducible representation. So, the direct product of two irreducible representations can also be turned into a unitary representations and now, we have the neat orthogonality property allowing us to decompose the direct product into a direct sum of irreducible representations (we're also using the property that for finite dimensional representations, if you keep taking proper subrepresentations, you'll hit an irreducible representation eventually. There's no infinite strictly decreasing sequence of positive integers). See Maschke's theorem.
If i≠j, then this decomposition does not contain the trivial representation (Otherwise, we'd have a nonzero intertwiner from Vj to Vi contradicting Schur's lemma). If i=j, then it contains exactly one copy of the trivial representation (Schur's lemma states that if A and B are two intertwiners from Vi to itself, since they're both multiples of the identity, A and B are linearly dependent). Therefore,
Applying a result of linear algebra to both orthogonality relations (|Ci| is always positive), we find that the number of conjugacy classes is greater than or equal to the number of inequivalent irreducible representations; and also at the same time less than or equal to. The conclusion, then, is that the number of conjugacy classes of G is the same as the number of inequivalent irreducible representations of G.
Corollary. If two representations have the same characters, then they are equivalent.
Proof. Characters can be thought of as elements of a q-dimensional vector space where q is the number of conjugacy classes. Using the orthogonality relations derived above, we find that the q characters for the q inequivalent irreducible representations forms a basis set. Also, according to Maschke's theorem, both representations can be expressed as the direct sum of irreducible representations. Since the character of the direct sum of representations is the sum of their characters, from linear algebra, we see they are equivalent.
We know that any irreducible representation can be turned into a unitary representation. It turns out the Hilbert space norm is unique up to multiplication by a positive number. To see this, note that the conjugate representation of the irreducible representation is equivalent to the dual irreducible representation with the Hilbert space norm acting as the intertwiner. Using Schur's lemma, all possible Hilbert space norms can only be a multiple of each other.
Let ρ be an irreducible representation of a finite group G on a vector space V of (finite) dimension n with character χ. It is a fact that χ(g) = n if and only if ρ(g) = id (see for instance Exercise 6.7 from Serre's book below). A consequence of this is that if χ is a non-trivial irreducible character of G such that χ(g) = χ(1) for some g≠1 then G contains a proper non-trivial normal subgroup (the normal subgroup is the kernel of ρ). Conversely, if G contains a proper non-trivial normal subgroup N, then the composition of the natural surjective group homomorphism G → G/N with the regular representation of G/N produces a representation π of G which has kernel N. Taking χ to be the character of some non-trivial subrepresentation of π, we have a character satisfying the hypothesis in the direct statement above. Altogether, whether or not G is simple can be determined immediately by looking at the character table of G.
History
The general features of the representation theory of a finite group G, over the complex numbers, were discovered by Ferdinand Georg Frobenius in the years before 1900. Later the modular representation theory of Richard Brauer was developed.
Generalizations
The Peter–Weyl theorem extends many results about representations of finite groups to representations of compact groups.
See also
- Character theory
- Real representation
- Representation theory of the symmetric group
- Schur orthogonality relations
- Deligne–Lusztig theory
References
- Fulton, William; Harris, Joe (1991), Representation theory. A first course, Graduate Texts in Mathematics, Readings in Mathematics, 129, New York: Springer-Verlag, ISBN 978-0-387-97495-8, MR1153249, ISBN 978-0-387-97527-6
- The standard graduate level reference for representations of groups in general, particularly Lie groups.
- James, Gordon; and Liebeck, Martin (1993). Representations and Characters of Finite Groups. Cambridge: Cambridge University Press. ISBN 0-521-44590-6.
- A beautiful and readable introduction; designed for self study.
- Serre, Jean-Pierre (1977). Linear Representations of Finite Groups. Springer-Verlag. ISBN 0-387-90190-6.
- A very well-written introduction to stated topic: concise and extremely readable.
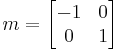
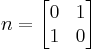
![f''(x\otimes y)=\sum_{g\in G}\langle x,\rho(g)[y]\rangle g](/2012-wikipedia_en_all_nopic_01_2012/I/1c824075db156c2c52c710790bea445a.png)
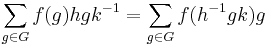
![f''(X\otimes Y)=\sum_{g\in G}\langle X,\rho(g)[Y]\rangle g=\sum_{g\in G} \langle X,Yg^{-1}\rangle g](/2012-wikipedia_en_all_nopic_01_2012/I/e50554747966fe83807e6efcebf6dd8d.png)